Unlocking Real-World Wonders: The Power of Trig Application Problems
Have you ever looked at a tall building and wondered how its height is measured? Or perhaps you've been intrigued by the intricate angles and distances involved in navigating a ship or an airplane? These real-world wonders, and countless others, can be understood and even solved using the power of trigonometry. While many may associate trigonometry with abstract formulas and equations, its true beauty lies in its ability to explain and solve practical problems that surround us. This is where "trig application problems" come into play, serving as the bridge between theoretical knowledge and real-world applications.
Imagine trigonometry as a toolbox filled with specialized tools. You've got your sine, cosine, tangent, and other trigonometric functions, each designed for a specific purpose. Now, think of trig application problems as the blueprint and instructions that guide you on how to use these tools effectively. They present you with realistic scenarios—be it calculating the angle of a ramp, determining the distance across a lake, or even analyzing the trajectory of a projectile—and challenge you to apply your trigonometry skills to find solutions.
The roots of trigonometry can be traced back to ancient civilizations like the Babylonians and Egyptians, who utilized its principles for astronomy, surveying, and architecture. However, the development of trigonometry as a distinct branch of mathematics is often credited to ancient Greek mathematicians. Hipparchus of Nicaea, often hailed as the "father of trigonometry," is known for his work on creating the first trigonometric table. This laid the foundation for centuries of further development and refinement of trigonometric concepts and applications.
The importance of trig application problems worksheets lies in their ability to bridge the gap between theory and practice. While textbook exercises might focus on memorizing formulas and solving equations in isolation, application problems challenge students to think critically, visualize situations spatially, and apply trigonometric principles in a meaningful context. They provide a practical framework for understanding why trigonometry matters in fields ranging from engineering and physics to architecture, navigation, and even music!
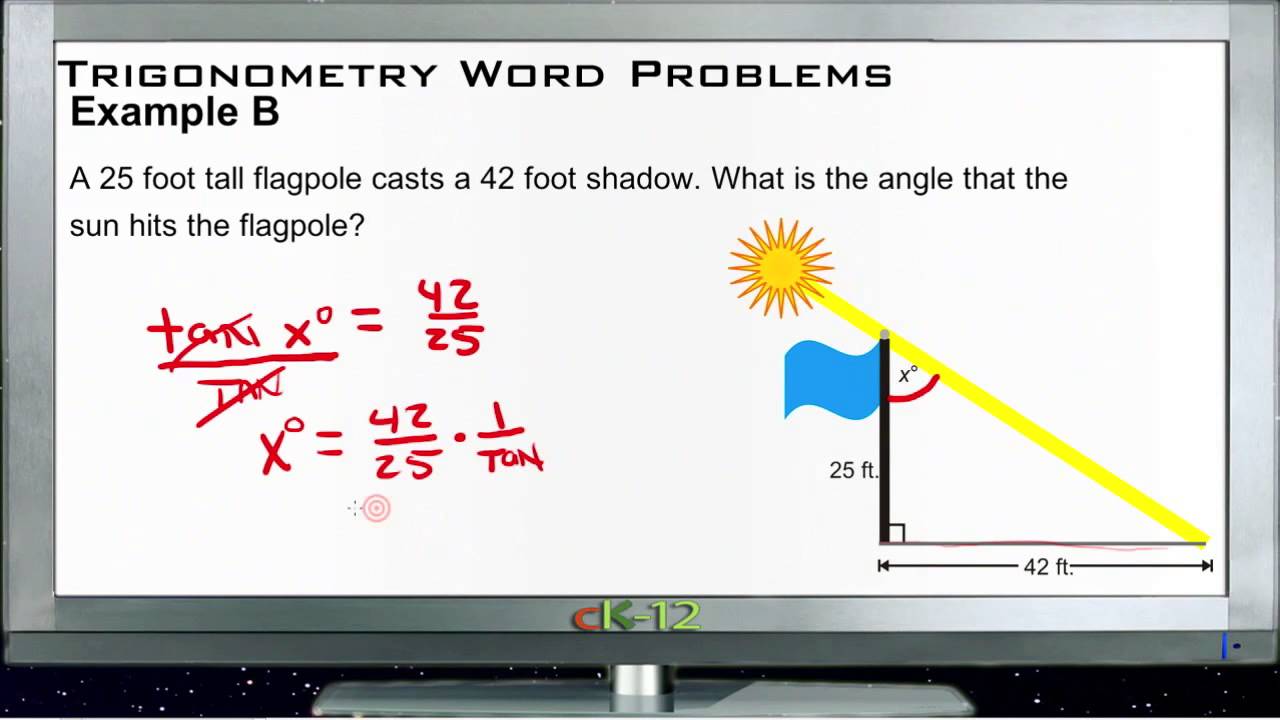
One common issue encountered by students tackling these problems is the struggle to translate word problems into accurate diagrams and equations. The ability to visualize the problem, identify the known and unknown variables, and then select the appropriate trigonometric ratios are crucial skills that require practice and guidance. This is where well-designed worksheets, interactive online tools, and clear explanations from teachers play a vital role in scaffolding student learning and building their problem-solving confidence.
Let's dive into a simple example to illustrate the practicality of trig application problems. Imagine you're standing at a point on the ground, looking up at a hot air balloon floating gracefully in the sky. You know the distance from where you stand to a point directly below the balloon is 50 meters. You also estimate the angle of elevation—the angle between your line of sight and the horizontal—to be 30 degrees. Using this information and a bit of trigonometry, you can actually calculate the height of the balloon!
Finding comfort exploring the beauty of frases bonitas de difuntos
Finding peace in kampar your guide to asar prayer times
Unlocking clayton county your guide to the georgia government website